1. INTRODUCTION *

2. BASIC CONCEPT OF GAME THEORY *

2.1 Prisoners' Dilemma *

2.2 Normative Properties of Dominant Strategy Equilibrium *

2.3 Implications for the Emergence of Cooperative Behaviour *

3. MAINTAINING COLLECTIVE ACTION - A ROLE FOR GOVERNMENT? *

3.1 Problems Associated with Market Failures *

3.2 Problems Associated with Collective Action *

4. A BRIEF ANALYSIS INTO ARMS RACES *

4.1 Modelling the Nuclear Arms Race in the Indian sub-continent *

5. LIST OF REFERENCE *

6. APPENDICES (News COVERAGE ON INDIA & PAKISTAN'S NUCLEAR TESTS) *

There was this story I read from a magazine few years ago which seems fictitious, but it makes a profound point in the analysis of strategies:

A music conductor was on his way from Leningrad to Moscow by train. Two KGB agents who had been watching his movements detained him while he was studying a musical score. They reckoned that those funny musical notes in the score are secret coded messages and charged him a spy. "Hey, those are notations from Tchaikovsky's Symphony No. 5!" he insisted. "We've caught Tchaikovsky too, and he's already talking…now confess and tell me about him!" yelled one KGB agent.

What should the conductor do? He knows the Soviet secret police's S.O.P.:

If he "confesses" and implicate his "collaborator", he will get off with a year in prison while "Tchaikovsky", or whoever he may be, will get 20 years in Siberia! But if "Tchaikovsky" confesses while the conductor maintains his innocence, the sentences will be reversed. However if both confess, each will get 10 years; or two years each if both maintain their innocence, as the KGB lacks confessions to press charges.

This is prisoners' dilemma, and so are many players' who are involved in strategic decision-makings! In the world of competition and survival, many organisations (and individuals) are confronted with decisions that must take into account more explicitly for the rival's likely responses. We can model this by a non-zero sum (and often non-cooperative) game like the prisoners' dilemma.

This essay will illustrate the dominant strategy adopted by each player in a two-person non-zero sum game, and outline the normative properties of the equilibrium and its implications for the emergence of cooperative behaviour between economic agents. The role of government as a mechanism for maintaining collective action will also be discussed. In our discussion, I will draw on the problems of arms races to analyse using the prisoners' dilemma.

2.    BASIC CONCEPT OF GAME THEORY

Game theory, as defined by Danben (1996), is a branch of mathematical analysis developed to study decision-making in any situation involving a conflict of interest. In such a "game", two or more decision-makers or "players" will select an optimum strategy in the face of an opponent who has a strategy of his own, leading to a desired outcome.

Game theorists have identified many types of games. In zero-sum games, the players have exact opposite interests, thus the total amount won is exactly equal to the amount lost (Danben 1996). In non-zero sum games, they have some interest in common. When the players can agree on a plan of action, they are in a cooperative game. In a non-cooperative game, the players cannot coordinate their choices. Coordination may be impossible if the players cannot communicate, if no institution exists to enforce an agreement, or if coordination is forbidden by law.

2.1    Prisoners' Dilemma

In the scope of microeconomics, the most relevant game is the prisoners' dilemma. This game provides some essential features of oligopoly market structure, and offers a good illustration of how it leads to predictions about the behaviour of decision-makers in competing for a larger share of profit amongst competitors. Similarly, in our analysis of arms races amongst nations, the players would face the same dilemma of choosing the best strategy taking into account the tactics adopted by its opponent.

In the case of the poor conductor and "Tchaikovsky" illustrated earlier, we can draw up a set of strategies and payoffs for both "prisoners" represented by the payoff matrix overleaf:



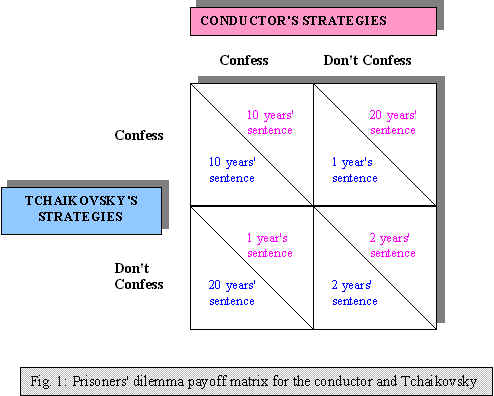
The strategies in this case are: Confess or Don't confess. The payoffs (penalties actually!) are the sentences served. How to solve this game? What strategies are "rational" if both men want to minimise the time they spend in jail? The conductor might reason this way:

"Two things can happen: Tchaikovsky can confess or keep quiet. Suppose Tchaikovsky confesses, then I'll get 20 years if I don't confess, or 10 years if I do -- so in that case it's best to confess. On the other hand, if he doesn't confess, I'll get 2 years if I don't either, but in that case, if I confess I'll get only a year! Either way, it's best if I confess…"

But Tchaikovsky can, and presumably will reason in the same way -- so they both confess and go to the prison for 10 years each. Yet, if they had acted "irrationally" maintaining their innocence, they could have gotten off with 2 years each. In this game, to confess is a dominant strategy and when both prisoners confess, that is the dominant strategy equilibrium and also a Nash equilibrium (at quadrant I).

 

2.2    Normative Properties of Dominant Strategy Equilibrium

The prisoners' dilemma illustrates how self-interest may lead to a world of non-cooperation. Here we assume that there is no communication between the two "prisoners". However, if they could communicate and commit themselves to coordinated strategies, they are unlikely to fall into quadrant I but end up in quadrant IV where both are better off.

If this game is played repeatedly, players are also more likely to arrive at some form of cooperation after developing their reputations about their behaviour, and studying the behaviour of their opponents. One strategy that worked best is "tit-for-tat", which means that what each player should do this round depends on what the other players did on the previous round. According to McTaggart, Findlay & Parkin (1996, p. 315), a trigger strategy may occurs as the other extreme end of punishment indicating there are "other intermediate degree of punishment". One example could happen if one player cheats on one period, the other player could punish by refusing to cooperate for a certain number of periods.

The more important feature of the DSE (or the Nash equilibrium for that matter) is that the outcome (or payoffs) is not what the players would unanimously wish for. This remarkable result -- that individual rational action results in both players being made worst off in terms of their own self interested purposes -- is what has made the wide impact in modern social and political science.

It should be noted, however, that DSE (as well as Nash equilibrium) may be unique, exist in multiple (ie. more than one DSE), or does not exist at all.

 

2.3 Implications for the Emergence of Cooperative Behaviour

We have discussed how a possible cooperation between players of a prisoners' dilemma game will lead to a Pareto efficient outcome. But is this outcome socially optimal? To give a better picture, let's reinterpret the above prisoners' dilemma game to one of the business world. Assuming 2 rival airline companies -- Quantas domestic & Ansett, fighting for a bigger market share for domestic air routes between Perth and Melbourne or Sydney. The variables in this game is the output choice (ie. the number of seats) manipulated by the price setting, ie., setting a higher price for the airfare results in a lower output, and vice versa. If we represent this game in an extensive form, we have the decision tree below:



 

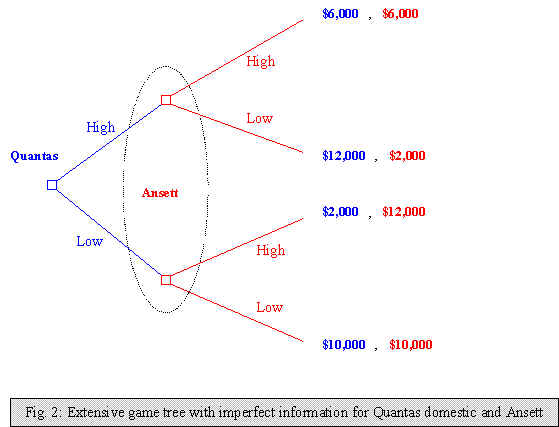
Here, we assume that both airlines have imperfect information, illustrated by Ansett ignorance about which decision node it was in before making its move. If both airlines were to adopt their dominant strategy, the dominant strategy equilibrium would be for both to choose high output (competing with lower prices to undercut each other) and earn $6,000 each.

However, this DSE is Pareto inefficient, as both could be better off if they cooperate and both produce low output (by raising their prices simultaneously) to rip off a higher profit from consumers (making $10,000 each). Although this cartel-like behaviour could lead to Pareto efficient outcomes for both airlines, these outcomes are not social optimal. This is due to the fact that these firm-desired outcomes are achieved by firms fixing prices above marginal cost and restricting output to achieve full cartel outcome, which is not socially desirable.

It should be noted that even this price fixing practice is legal, there is an incentive to cheat which would limit the participants from achieving the full cartel outcome. Referring to the normal form of the above game illustrated below, either airline has the incentive to adopt high output, and if the other remains at agreed-upon level of output (producing low in this case). The cheating firm will earn $12,000 while the other gets only $2,000. However if both firms cheat, the collusion will breakdown with both firms reverting to their dominant strategy of producing high and earning $6,000 each (quadrant I).



 

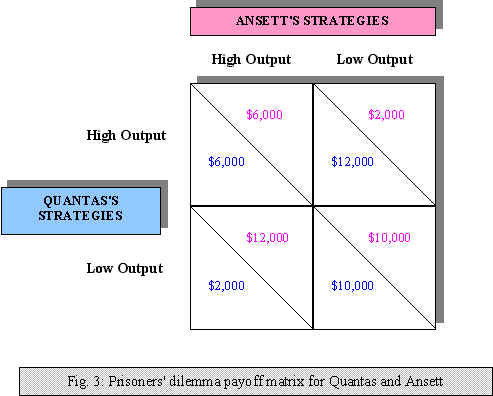
3.    MAINTAINING COLLECTIVE ACTION - A ROLE FOR          GOVERNMENT?

As illustrated in the previous section, if firms collude and form cartels to fix price and limit output, it is not social optimal. By doing so, Katz & Rosen (1994, p. 420) pointed out that the necessary condition for Pareto efficiency is violated, and thus the First Fundamental Theorem of Welfare Economics does not hold.

The First Welfare Theorem states that "a competitive economy with a market for every commodity generates a Pareto-efficient allocation of resources without any government intervention [emphasis added]" (Katz & Rosen 1994, p. 421). But in reality, the required competitive environment does not always exist: monopolies, cartels, and to a lesser extend, oligopolies and other market structures that have a certain degree of market power can cause market failure.

The role of government to correct market failure is thus to enhance efficiency. The government could do this by breaking monopolies and outlaw formation of cartels in order to limit market power and restore a social optimal outcome.

In America, the Antitrust laws was enacted with the primary objective "to promote a competitive economy by prohibiting actions that restrain, or are likely to restrain, competition, and by restricting the forms of market structure that are allowable" (Pindyck & Rubinfeld 1989, p. 363); while in Australia, the Trade Practices Commission act against certain restrictive practices (such as collusive pricing and retail price maintenance, through the Trade Practices Act, 1974) but it does not assume that market concentration necessarily means market power and behaviour contrary to consumer interests (Samuelson et al 1992, p. 326-27).

On the other hand, Hardin (1971) have shown using a n-person prisoners' dilemma that collective action of large number of players in contributing toward the purchase of the group collective interest would fail with each player defecting from contribution and played the dominant strategy in a "distrust" environment.

In this case, the government can play a role in ensuring all players contribute toward the objectives by imposing "sanctions" or providing private benefits to participating members in the form of "injunctive proscription, prescriptions, or fines and subsidies" (Ordeshook 1986, p. 224) as proposed by Olsen (1968).

The government's environmental protection policies that imposed fine to those found polluting the environment is one example of government's effort to maintain collective action.

Anderton (1986, p. 9) defines an arms race to be "a situation where two or more parties change the quantity or quality of their armed forces in response to perceived past, current or anticipated future increases in the quantity or quality of armed forces of the other party(ies)". This definition includes situations where each nation reacts positively to the other and in particular increases its military expenditures when the other does. According to Isard (1988), each may do so in order to:

1. reduce insecurity (reflecting a balance of power motivation);
2. retaliate for the insecurity caused by the other's increased military expenditures and military activities (reflecting a balance of terror motivation);
3. counteract the ambition of the other; and/or
4. be in a position of greater strength when negotiations on arms control are expected in the future.

In our analysis, we shall focus on the recent stand-off between the Indian and Pakistan government caused by their nuclear testing programmes.

4.1    Modelling the Nuclear Arms Race in the Indian sub-continent

India has fought three wars with Pakistan since 1947 and suffered a crushing defeat at the hands of China in a 1962 border conflict. The following year, it denied an allegation by the U.S. that it produces an atomic bomb to counter China, which was expected to become a nuclear power. It was only after Pakistan's commenced its alleged clandestine nuclear weapons program in 1972 that India took the unavoidable counter-measure of conducting its first nuclear test in Pokhran, in 1974 (Kapur & Wilson 1996; Moshaver 1991).

Before those developments, the dilemma then facing both would-be nuclear nations were whether to engage in the development of nuclear weapons or not: a classic gun verses butter issue.

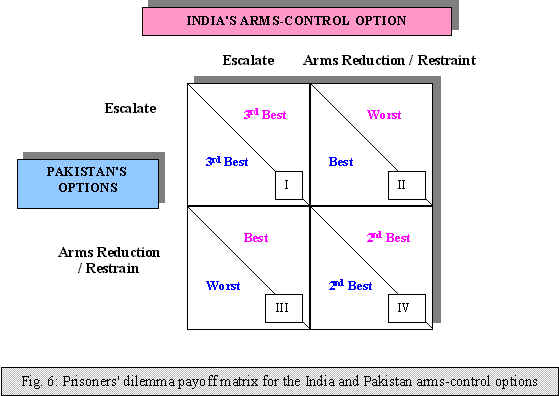
We can model this vaguely in a prisoners' dilemma payoff matrix overleaf:

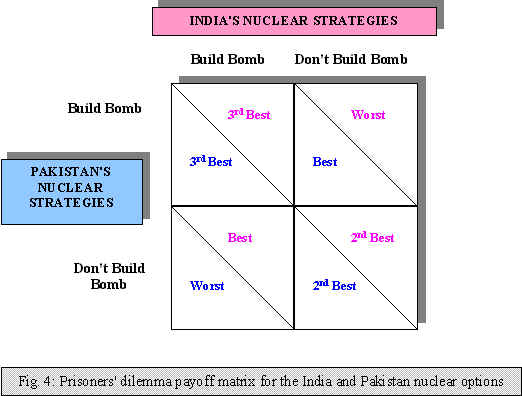
Here, both nations identified the advantage of possessing nuclear weapons if the other does not. Both nations, being non-signatories of the nuclear Non-Proliferation or other related treaty, cannot rely on each other's assurance to stay in quadrant IV, where both "don't build bomb" and concentrate on their economic development, deemed second best option for both. Consequently, they ended up in the DSE.

"If India openly starts a weapons programme, the deep-rooted Pakistani fears of India…would put tremendous pressure on Pakistan to take appropriate measures to avoid a nuclear Munich at India's hands in the event of an actual conflict, which many Pakistanis think very real."

Senior Pakistani nuclear scientist,

Abdul Qadir Khan, Fall 1985

"If Pakistan gets a nuclear weapon, India will have to think very seriously about its own option. …We have every indication and information that leads us to believe that Pakistan has not given up its nuclear weapons program and is bent on having it."

The late Indian Prime Minister,

Rajiv Gandhi, April 1986

The second Indian nuclear test of 5 atomic devices 24 years later on the 11 and 13 May 1998 came after the fact that Pakistan has not only acquired nuclear weapons and missile capability, but also her Prime Minister threatened their use against India (Asiaweek 1998).

Then came the dramatic announcement of Pakistan's first-ever nuclear tests on 29 May followed by another one the following day bringing a total of six devices exploded. This matches India's total since 1974.

''Today we have successfully carried out five tests...we have evened the account with India"

Pakistan Prime Minister

Nawaz Sharif, 29 May 1998

''We did not force Pakistan into this. In fact, it is Pakistan's clandestine preparations forced us to the path of nuclear deterrence"

                                                                                                                                            Indian Prime Minister

                                                                                                                                     Atal Bihari Vajpayee, 29 May 1998

The 6 nuclear devices detonated by Pakistan portrayed Pakistan's desire to match India's nuclear capabilities at least in quantity. If we assume that India wanted to lead Pakistan in terms of the number of nuclear arsenal by, say 10% as her national security policy, we can represent the dynamics of this nuclear weapons build-up in the diagram below:

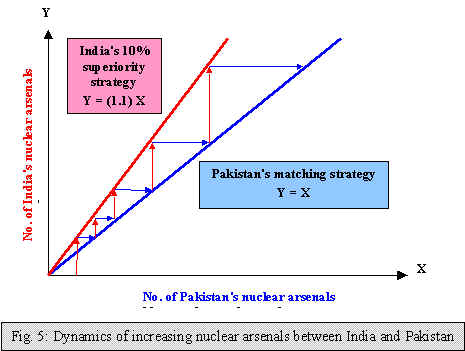
The vertical red arrows show India's increment of its nuclear weapons to keep up her national security policy of 10% superiority over her rival; while the horizontal blue arrows show Pakistan's desire to match India's number every round. This can leads to an endless arms race.

"As a responsible nation, whose record of restraint and responsibility is impeccable, Pakistan today assures the international community and, in particular, India, of our willingness to enter into immediate discussions to address all matters of peace and security, including urgent measures to prevent the dangers of nuclear conflagration"

                                                      Pakistani Foreign Secretary,

Shamshad Ahmad, 30 May 1998

 

 

With the latest indication by India and Pakistan to go to the negotiation table, we would expect to see some form of arms control. Again, we can model this situation below:

If both nations can work together closely to abide by the arms control agreement (without attempting to cheat), we can expect to see a peaceful solution to the tension in the Indian subcontinent with both India and Pakistan refraining from escalating the nuclear arms race.

Although game theory can be (and have been) used to analyse political and military events, they have been criticised as a dehumanising and potentially dangerous oversimplification of necessarily complicating factors (Dauben 1996) which would lead to deficiency in their analysis.

 No. of words: 2496 (Contents Only)

 5. LIST OF REFERENCE

Anderton, Charles H. (1986) 'Arms Race Modelling: Systematic Analysis and Synthesis', Ph.D. dissertion, Cornell University.

Asiaweek (1998) 'Why India's Bomb is Justified' Asiaweek Online, Accessed on 24 May 1998 at [http://www.pathfinder.com/asiaweek/current/issue/nat3.html]

Axelod, Robert (1984) The Evolution of Cooperation, Basic Books, New York.

Danben, Joseph Warrant (1996) 'Game Theory', in Microsoft ® Encarta ® 97 Encyclopedia, Microsoft Corporation.

Hagenmayer, J., and Glazer, A. (1992) 'Albert W. Tucker, 89, Framed Mathematician', Philadelphia Inquirer, 2 Feb, p. B7.

Hardin, R. (1971) "Collective Action as an Agreeable n-Person Prisoners' Dilemma', Behavioural Science, 16. p. 472-81.

Isard, Walter (1988) Arms Races, Arms Control, and Conflict Analysis, Cambridge University Press, New York.

Kapur, Ashok (1996) Foreign Policies of India and her Neighbours, St. Martin's Press, Inc., New York.

Katz, Michael L. and Rosen, Harvey S. (1994) Microeconomics, 2^nd Ed., Richard D. Irwin, Inc., Illinois.

McTaggart, D., Findlay, C., and Parkin, M (1996) Microeconomics, 2^nd Ed., Addison-Westley Publishing Co., Sydney.

Moshaver, Ziba (1991) Nuclear Weapons Proliferation in the Indian Subcontinent, MacMillian Academic and Professional Ltd., Hampshire

Olsen, M. (1968) the Logic of Collective Action, Harvard University Press.

Ordeshook, P.C. (1986) Game Theory and Political Theory: An Introduction, Cambridge University Press.

Pindyck, R.S., and Rubinfeld, D.L. (1992) 'Market Power: Monopoly and Monopsony' in Microeconomics, 2^nd Ed., MacMillian Publishing Co., New York.

APPENDICES (News Coverage on India & Pakistan's Nuclear Tests)

Period of Coverage : 24 - 31 May 1998

Sources :

• Straits Times Interactive [http://www.asia1.com.sg/straitstimes/]
• The Times of India Online [http://www.timesofindia.com/today/]
• The News Pakistan [http://www.jang-group.com/thenews]
• Asiaweek Online ;www.pathfinder.com/asiaweek/current/issue]

Return to Assignments for Semester 1, 1998